
Problem 4a, b, c: How many significant figures do each of the centimeter measurements have?
| Problem 1: When is a number never itself? |
| Problem 2a, b, c: How many significant numbers do each of these 3 decimeter measurements have? |
 |
| Problem 3a, b, c: What are the 3 decimeter measurements above when written in centimeters? (Note: The centimeters may have to be written in scientific notation in order to reflect the correct significant figures). Problem 4a, b, c: How many significant figures do each of the centimeter measurements have? |
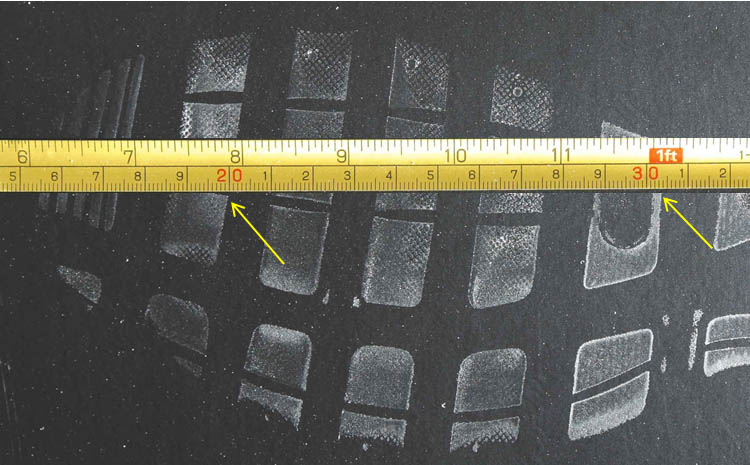
| Problem 5: The below image is that of a shoe print at a crime scene. The ruler has inches on the top and metrics on the bottom. Which would give you more accuracy in measuring and why? | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
 |
Problem 6: This is one end of an architect ruler. At the top between the 1/8 and "0" are small divisions. The distance between the long line on the left and the "0" is 1/8 of an inch. What fraction of an inch are the smallest divisions?
Problem 7: At the bottom is a zero and 1/2. That one half inch has been divided up into smaller divisions. What fraction of an inch is the smallest divisions? |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
 |
It's hard to read but below the display, the balance has the words "150g d=0.01g". The "150g" is the maximum grams this balance can weigh. The "0.01g" is the accuracy. So you could measure something that was 150.00 grams. Problem 9: How many significant figures is that? Problem 10: If you used this balance to measure a nickel, you might get 5.02 gram answer. How many significant figures is that? Problem 11: If you used this balance to weigh 25 nickels, you might get 125.13 as the gram weight. How many significant figures is that? Problem 12: Divide the 125.13 by 25 to get the average mass of a nickel. Report the answer to the proper number of significant figures. |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
 |
Problem 13: One lab partner weighed some powder and wrote down 45 grams as its mass. The other lab partner weighed more of the same powder and wrote down 43.15 grams as the mass. The two samples were then combined. Without weighing the combined sample, what would you say their combined mass would be?
Problem 14: Another group had one person measure the powder and wrote down 34.70 grams. Another person in the group measured more powder and recorded 32.08 grams. The two powder samples were combined. What would you record for their total mass? |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
 |
Problem 15: Using money is a good lesson in significant figures. One friend says he has $20. Another says he has $30, and a third says he has $40. All of these have only one significant figure each, meaning they rounded their money to the nearest $10. So what is the lowest total amount that this group could have? Problem 16: What is the highest total amount that this group could have? |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In the table below, the calculations are done but they need to be rounded to the proper number of significant figures. Problem 17: What is F2 in the correct number of significant figures? (Note: the 1mL is considered exact) Problem 18: What is F6 in the correct number of significant figures? Problem 19: What is F10 in the correct number of significant figures? (Note: the 100mL in the concentration is considered an exact amount.) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||