Balancing Chemical Equations
Chemical Equations vs. Math Equations
60 =
20 + 8x
H2CO3
-> H2O + CO2
Reactants ->
Products
When you see the name "chemical equations,"
the word "equation" makes us think of math equations. There
is some similarity. Like all equations, something has to be "equal."
In a math equation the value of the left side of the equal sign is the
same value as the right side. These values also exist at the same time
like a balance.
In a chemical equation the atoms on the left side have to be equal to
the atoms on the right side of the arrow, but they don't exist at the
same time. The chemical equation represents movement. For example, here
we start with carbonic acid consisting of 2 hydrogen atoms, 1 carbon atom,
and 3 oxygen atoms. The atoms rearrange and become water and carbon dioxide.
All original atoms are still accounted for.
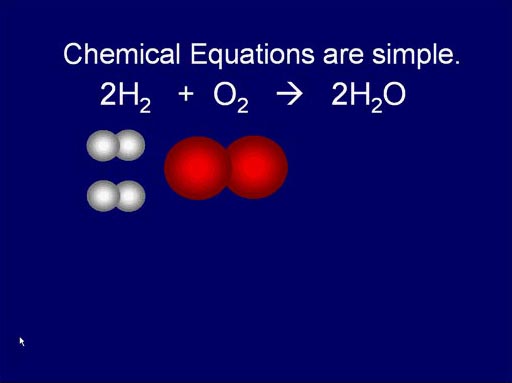
Chemical Equations are simple:
Like I said earlier, atoms combine in simple ratios. That
means the chemical equations that show and balance the starting "reactants"
and ending "products" are fairly simple. Unlike math equations,
chemical equations are showing a progression of time.
Here we see how hydrogen and oxygen (reactants) react and produce water.
It's a simple rearrangement.
Roll mouse over image to see animation.

Bike Example for Balancing Chemical Equations:
We are all familiar with bicycles, tricycles, etc. It's also easy to see how many wheels are necessary to build them. Because this is very visual (and atoms are too small) I'm going to use the manufacturing of these products as an introduction to balancing chemical equations.

This company is going to promote their super durable tires. The tires will be the biggest expense in making these cycles. Because of that, the company may recall cycles that aren't celling and put the wheels on the cycles that are selling.
Notice that the wheels are only sold to the manufacturer in pairs.

The company that produces these pedal cars can use abbreviations similar to the way chemistry uses abbreviations. For example Cb can represent the "Car body". "W" will represent the "wheels." Remember that the wheels are only purchased in pairs. So "W2" repesents a pair of wheels. The production scheme is that one car body (Cb) is added to two pairs of wheels (2W2) to make a complete car (CbW4).
We know a car has four wheels, so the formula of CbW4 should seem logical.
The approach in balancing chemistry equations uses the same logic.
We
need fire extinguisher fluid (carbon tetrachloride)
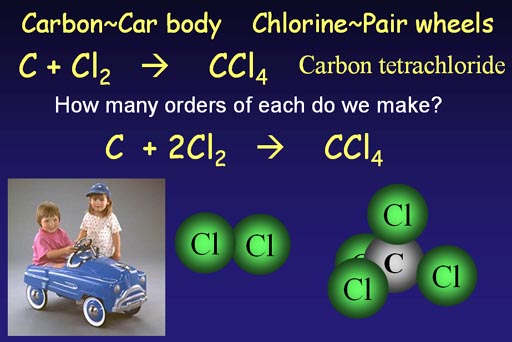

The carbon "C" is like the car body. Chlorine always comes as a pair, so it's like the wheels that come in pairs.
To make carbon tetrachloride we need carbon and chlorine.
C + Cl2 -> CCl4
This is the unbalanced chemical equation because the number of chlorine
atoms in the product is different than what we started with. We are 2
chlorine atoms short, so we show two pairs of chlorines.
C + 2Cl2 -> CCl4
This is the balanced chemical equation.

When we manufacture tricycles, we run into a problem. We know we need to add wheels (W2) to a trike body (Tb). This gives us the unbalanced equation:
Tb + W2 -> TbW2
As you can see this number of wheels don't match. If we use two pairs of wheels (2W2), we end up with an extra wheel. So we compensate by starting with two trike bodies. Then we are short wheels, so we go with 3 pairs. At this point we the number of trike bodies and wheels we start with match what we end with; so it's now a balanced production equation.
Making aluminum chloride
(anti-perspirant ingredient)
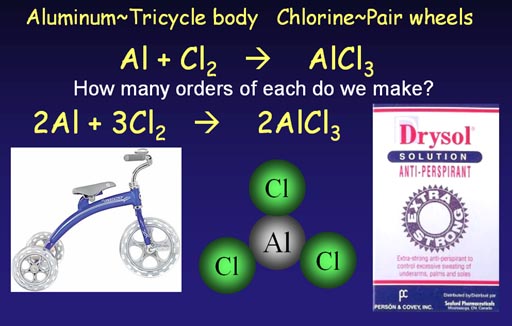
(anti-perspirant ingredient)

A trike body attaches to 3 wheels. Aluminum attaches to
3 chlorine atoms. Because of this similarity, the equation for making
aluminum chloride has the same issues. We start with an unbalanced (skeleton)
chemical equation.
Al + Cl2 -> AlCl3 (short 1 chlorine at start)
Al + 2Cl2 -> AlCl3 (ends up with extra
chlorine)
2Al + 2Cl2 -> 2AlCl3
(short 2 chlorines at start)
2Al + 3Cl2 -> 2AlCl3 (all atoms match)
We see that starting with Cl2 is not enough chlorine atoms, so we go with 2Cl2 but then end up with an extra chlorine atom. So we go with 2 aluminum atoms, but then we are short 2 chlorine atoms. Finally, by going with 3 pairs of chorines atoms, we end up with a balanced equation.
Cars not selling, so use car wheels to make bicycles
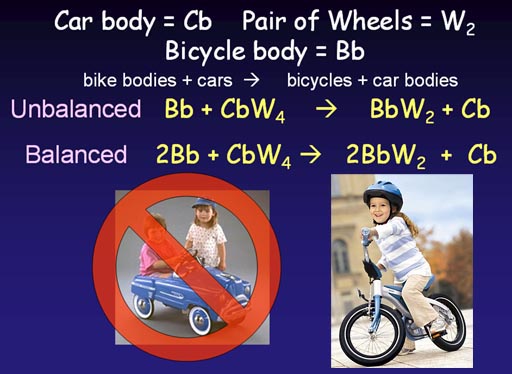
So far we've done a simple synthesis where one thing is
built from simpler pieces. Now we will do what's called a "single
replacement" or "single displacement" reaction. That's
when one part is replaced (displaced) with another. In this example, the
car body is being replaced with a bike body. The wheels are being reused.
In our unbalanced (skeleton) equation, we simply write that we start with
a bike body and car with wheels and end up with a full bike and car body
without wheels. Unfortunately the number of wheels don't match. A manufacturer
would go bankrupt if it doens't know how to get the proper number of starting
materials so that there's no leftover pieces at the end of the production.
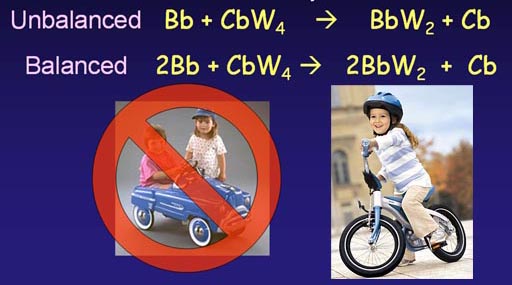
Looking at the unbalanced equation, we see that the number of wheels on the left side (4 wheels) are twice as many as those on the finished side (2 wheels). So we see that if we finished with 2 bicycles, we would use all 4 wheels. To finish with two bicycles we have to start with 2 bicycle bodies. Now we have a balanced equation.
Anti-perspirants not selling, we need
de-icers. Let's use the chlorines from one to make the other.

A chemistry example equivalent to the above example is changing carbon tetrachloride (fire extinguisher fluid) to calcium chloride (deicer powder).
Like the above example, the carbon tetrachloride has twice the chlorine atoms than the calcium chloride needs. This forces us to double the final calcium chloride compounds, which also means we must start with two calcium atoms. This is called a single replacement reaction because the carbon in carbon tetrachloride molecule gets replaced with a calcium atom.

3Ca + 2AlCl3 -> 2Al + 3CaCl2
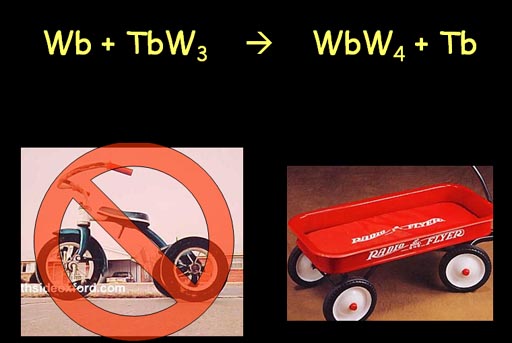
Here is another tricky one. We are taking tires off of trikes to make wagons. As you can see trikes has 3 wheels and wagons have 4 wheels. Let's see if you can do it. My only hint is 4x3=3x4.
Roll mouse over image to check your answer.
So far the wheels are simply transferred a different body. Now let's try some double swapping.

Let's say we have Three-wheeled fuselage [Tf] connected
to a rotor [R] (top image) and we want to take the wing off of a car fuselage
[CfWi] (bottom image) and make a winged 3-wheel fuselage [TfWi] (middle).
The car would then get the rotor (not shown): Here's the equation.
TfR
+ CfWi -> CfR
+ TfWi
Looking at this equation we see that the fuselages simply swapped places. The three-wheel fuselage that had a rotor now has a wing and the car fuselage that had a wing now has a rotor.
They call this a "double-replacement" or "double-displacement" reaction (no big surprise).
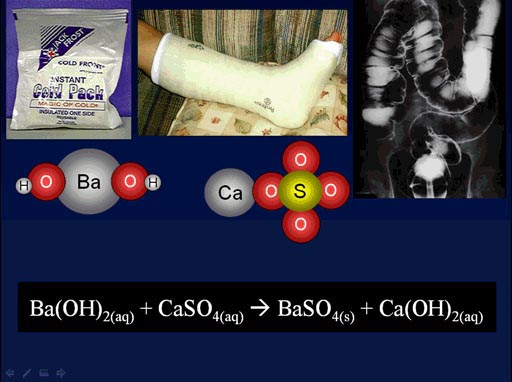
Here is a chemistry example that was shown in an earlier tutorial. This is also a double-replacement reaction.
Here the barium atom swaps places with the calcium atom. When that happens, we make barium sulfate which is given to patients to drink, so x-rays can see the stomach and intestines. As a kid I had to drink it, and it tasted like chalk. It was bad. They could have at least sweetened it.
(roll cursor over image to see the double-replacement animation)

The point I want to make with this tutorial is that the techniques of balancing the starting and ending amounts are useful to chemistry or any kind of production.
In a later tutorial, we will expand this analogy and deal
with handling large quantities of starting items (reactants) and products.
For now let's practice the balancing of somne equations.
Consider the balancing of the below equation:
H2 + O2 --> H2O
When balancing, you leave the formulas alone. In other words, H2, O2, and H2O will not change, just the number in front of them might change. You just try to figure out how many of each of these 3 will make what you start with the same as you end with.
?H2 + ?O2 --> ?H2O
Here's how we balance. We start looking at "H2" and realize that this is two hydrogen atoms. We then look at the right side of the equation (right of the -->). We notice that H2O also has 2 hydrogen atoms. That's good. We started with two hydrogen atoms and ended with 2 hydrogen atoms. So H2 + O2 --> H2O is balanced as far as the hydrogen atoms are concerned. We now look at the O2 and realize that this represents 2 oxygen atoms. We then look at the right side and notice that H2O only has one oxygen atom. That's not balanced because we don't lose or gain atoms when the equation is balanced.
To get 2 oxygen atoms on the right side, we have to write
H2 + O2 --> 2H2O. Notice that the left side has two oxygen atoms and the right side now has 2 oxygen atoms because if you have 2H2O (2 water molecules), there will be two oxygen atoms. Unfortunately, by writing 2H2O we just doubled the number of hydrogen atoms on the right side. In 2H2O, there are 4 hydrogen atoms. Now the hydrogen atoms don't balance because the starting side only has 2 hydrogen atoms (H2). To fix that, we write 2H2. So here's our final balanced equation.
2H2 + O2 --> 2H2O
Notice that if we count up hydrogen atoms to the left of the "-->", they match the number on the right side. The same is true for the oxygen atoms. Here's the count.
four H and two O --> four H and two O
H2SO4 + NaOH --> HOH + Na2SO4
This is a double replacement reaction and also called a neutralization reaction. It's a bit hard to see the double-replacement. If water is written as HOH, you can tell that the first H is the one that came from sulfuric acid and replaces the sodium (Na).
H2SO4 + NaOH --> HOH + Na2SO4
Here's how to balance this one. Start with the left compound (sulfuric acid) and look at the hydrogen. H2 means 2 hydrogen atoms. This balancing is a little tricky because these hydrogen atoms are what makes H2SO4 acidic. It's best not to mix these hydrogen atoms up with the one on NaOH.
On the right side there's only one "H" atom. So we balance it by putting a two in front of HOH
H2SO4 + NaOH --> 2HOH + Na2SO4
Right now, the acidic hydrogen atoms balance. So let's go on to the SO4 group (sulfate) in H2SO4 The left side only has one SO4 and the right side only has one. So the SO4 group is balanced. We then move on to "Na" in NaOH. There's only one sodium (Na) in NaOH, but on the right side Na2SO4 has two Na atoms. To fix this we need 2NaOH.
H2SO4 + 2NaOH --> 2HOH + Na2SO4
What's left is the OH (hydroxide) group. There's two on the left and two on the right, so those are balanced. So here's the before and after count:
2H, 1 SO4, 2Na, 2OH --> 2H, 2OH, 2Na, 1 SO4 They all balance. So the above equation is the balanced equation.
Here's how to balance this one.
Al2(CO3)3 --> Al2O3 + CO2
Start with the compound on the left side. We see that we begin with 2 aluminum atoms and end with 2 aluminum atoms. So the aluminum is balanced. Let's now look at the carbon atoms. In Al2(CO3)3 there are 3 carbon atoms. The right side shows only one carbon in CO2. So we write 3CO2.
Al2(CO3)3 --> Al2O3 + 3CO2
At this point aluminum and carbon atoms balance. We then check the oxygen atoms. In Al2(CO3)3 there are 9 oxygen atoms. Three are in CO3, but that is tripled, (CO3)3 So that gives us nine. On the right side there are 3 oxygen atoms in Al2O3 and 6 oxygen atoms in 3CO2 So that adds up to 9 oxygen atoms on the right side. That means the equation is balanced.
Al2(CO3)3 --> Al2O3 + 3CO2
Let’s start with balancing carbon atoms.
C3H5(NO3)3 -> 3CO2 + H2O + N2 + O2
When we try to balance hydrogen atoms we see that we have five on the left but hydrogen in water on the right is in pairs (H2O). So we have to double everything in order to balance 2x5 hydrogens on left and 5x2 hydrogens on right. We will also have to double the carbon atoms on left and right.
2C3H5(NO3)3 -> 6CO2 + 5H2O + N2 + O2
Now let’s balance the nitrogen atoms (Always balance oxygen last because it is in more than one compounds on the right). In (NO3)3, there are 3 nitrogen atoms, but that is doubled because of the 2 in front of nitroglycerin. So there are 6 nitrogen atoms on the left, which we can balance with 3 N2 molecules on the right.
2C3H5(NO3)3 ->6CO2 + 5H2O + 3N2 + O2
All that is left to balance are the oxygen atoms. The left side has 9 oxygen atoms in (NO3)3, but since there are two nitroglycerin molecules, that is doubled to make 18 oxygen atoms currently on the left side. On the right side we have 6CO2 + 5H2O + 3N2 + O2. The 6CO2 gives us 12 oxygen atoms, the 5H2O gives us 5 more, and then O2 is 2 more. That adds up to 19 oxygen atoms. So we are not balanced. It would be balanced if we just took ½ of O2 to get a single oxygen. Then the right side would have 18 oxygen atoms. Sometimes an equation might write ½O2 but that’s not normally wanted. So to get rid of ½O2, we just double everything.
4C3H5(NO3)3 -> 12CO2 + 10H2O + 6N2 + O2
Now we have 36 oxygen atoms on the left and 36 on the right. The other elements should still be balanced because they all got doubled equally.
By the way, the numbers in front of these compounds are called "coefficients". The "co" in coefficient means to "work with" such as in copilot. The "efficient" in "coefficient" means "make or do". So "coefficient" means a number that work with something else to make (or show) the final amount. For example, in "12CO2", the coefficient "12" works with CO2 to give us the total number of CO2 molecules.
2Cu(NO3)2 --> 2CuO + 4NO2 + O2
The hint is to do the oxygens last. Another hint is to start with the most complicated compound in the formula, which is Cu(NO3)2. Let's start with copper (Cu). We have one on the left and one on the right, so as far as copper is concerned, it is balanced. Now lets go to the "N" inside of Cu(NO3)2. There are two nitrogen atoms because of the parenthesis around the (NO3). So we have two N on the left side but only one in the NO2 on the right. So we make it 2NO2, so that the right side has two nitrogen atoms. So far we have this:
Cu(NO3)2 --> CuO + 2NO2 + O2
So far the Cu and the N balance. Now we tackle the oxygen at the end. The left side has 6 oxygen atoms in (NO3)2. The right side has 7 (CuO=1, 2NO2=4, and O2=2). So now our oxygen atoms on the left side are too few. The only way to increase them on the left side is to double the Cu(NO3)2 to 2Cu(NO3)2. Well, now we have to start over again. We have two copper atoms on the left so we have turn CuO into 2CuO, to get 2 Cu on the right.
The same for "N". We now have 4 "N" on the left from "2Cu(NO3)2". So we make the "2NO2" to "4NO2" in order to have 4 "N" also on the right. Our equation now is:
2Cu(NO3)2 --> 2CuO + 4NO2 + O2
Then we count the oxygen atoms again on the left. "2Cu(NO3)2" has 12 oxygen atoms. On the right side our "2CuO" gives us 2 oxygen atoms, the "4NO2" gives us 8. So that totals 10. The O2 makes 12 oxygen atoms, so we are now done. The O2 doesn't have to change.
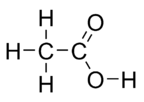
Summary of tips:
1) Start with the most complicated looking compound and balance the elements that are in that compound.
2) End by balancing oxygen (O2) last.
3) Never change the formulas. Just determine the number (coefficient) of each element or compound. (Exception: You can rewrite H2O as HOH when you have a chemical equation involving an acid or base. Another example would be organic acids (carbon based acids). You can put the acidic hydrogen in the front or at the end. For example, the formula for acetic acid is C2H4O2. This formula lumps all of the hydrogen atoms together. So you could rewrite it as HC2H3O2 so the first hydrogen is the acidic hydrogen which comes off. Or you could write it as CH3COOH. The end hydrogen is the one that comes off. Also, the COOH is the carboxylic acid group that is common to most organic acids. Below is the structural formula for acetic acid. Now you can see why it's written as CH3COOH.)